Semiotic Machines - an Introduction
Semiotic as a scientific Theory
AUTHOR: Dr. Gerd Döben-Henisch
FIRST DATE: Aug-6, 1996
DATE of LAST CHANGE: Sept-29, 1996
Working as a philosopher of Science I feel sympathetic with the semiotic approach of Charles Morris, at least with his foundations of 1938 (the citations will follow the edition of the Writings on the General Theory of Signs (1971) edited by Morris). In these writings he reveals strong influences of the main ideas of the philosophy of science of his time, especially of Carnap and Reichenbach.
In the Foundations he states clearly that semiotic should be a science, distinguishable as pure and descriptive semiotic (cf. Morris 1971: 17, 23), and that semiotic could be presented as a deductive system (cf. Morris 1971: 23).
In the time of Morris's writings the philosophy of science was dominated by what was later called the received view (for a good summary of the main lines of the discussion until 1967 see Suppe 1977), which has been primarily propagated by Rudolf Carnap.
Rooted in the Machean Neo-Positivism relying on sensations as data combined with the conventionalism of Poincar‚, the key-idea of the received view was, that a scientific theory has to use mathematics -or formal logic- as its general language to state regularities and common laws, but the terms of this mathematical language have to be interpreted by a clearly defined observational language, whose descriptive terms have to be based exclusively on observable phenomena (cf. SUPPE 1977:50ff).
During the fifties and sixties it became clear that this concept is unsatisfying. One of the main reasons for this was the fact that the theoretical terms at best can only partially be interpreted by the observational language. After the historical conference in Urbana (Illinois) in March 1969 the enthusiasme for the theoretical debates of philosophy of science cooled down a bit and until today we have no new single unifying view telling us how a scientific theory should be shaped.
For my proposal I will adopt ideas from the theory-concept as it has been pushed forward by the theoretical physicist Ludwig (1978, 1978b), by the structuralist view as it is explained in Wolfgang Balzer (1982) as well as in Balzer, Moulines, and Sneed (1987), and by the metatheoretical clarifications of Peter Hinst (1996). All these positions together have their roots in the structural concept of Bourbaki (1970). To distinguish my proposal from the cited ones I call my concept Minimal Theoretical Framework [MTF]. The intention of the Minimal Theoretical Framework is to allow as much cases of scientific activities as possible to be subsumed under this framework, including semiotic.
The main differences between the received view and the one used here are the following two: (i) the elements of the new theory concept are embedded in a pragmatic framework which allows a more general application of this concept, also in the case of non-empirical sciences; (ii) the relationship between the theoretical structure of the theory with the possible sets of data is relaxed to the postulate, that all protocol-statements have to be axioms of the theory and that no inconsistency is allowed.
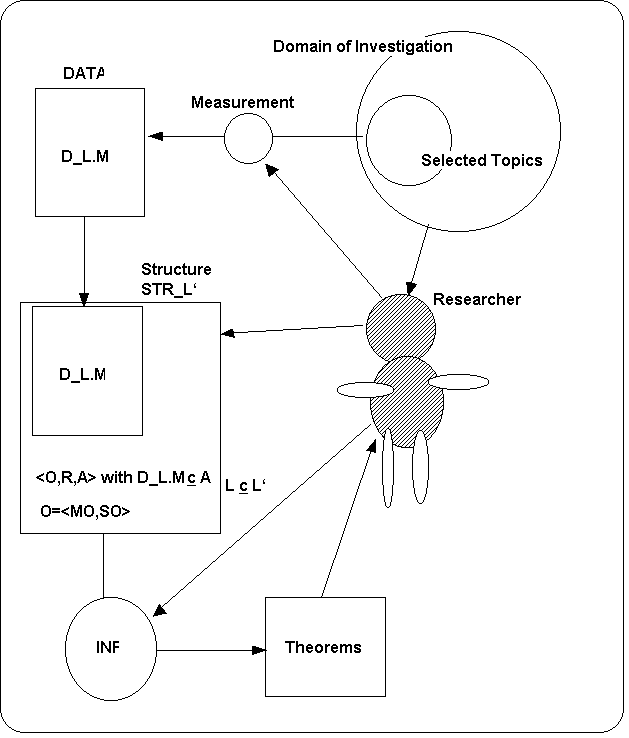
The Minimal Theoretical Framework presupposes science to be a discourse with humans as the main actors in the process of investigation. A participant of such a discourse is called a scientist. The following assumptions are made:
Any scientific discourse has to establish some domain of investigation. The selection of topics will be introduced by the definition of some method of measurement. A measurement will here be understood as a process which generates a mapping between elements of a supposed domain of investigation and descriptive statements. The communication of descriptive concepts demands for some kind of a language to represent the data of the intended theory. We call such a language the data language L. The text written with the data language is called a data protocol or simply the DATA. A single statement of DATA we call a datum [DAT].
The data protocol reports only single events, eventually indexed by some space and time notation. To represent complex relations between those events and to state general laws one needs an additional text STR which represents a formal structure, written in some language L'. The formal structure STR is organized as a tupel containing sets of objects O, subdivided into main objects MO and subsidiary objects SO, sets of relations R, and axioms A. The inference concept INF, which tells us what is a valid consequence of A, can be part of the presupposed set theory -which is assumed here-, or has to be individually introduced as part of the structure.
The combination of methods of measurement, data-protocols and a formal structure yields a scientific (explaining) theory T. It is assumed that all expressions of the data language L are also expressions of the theory language L' and that L' and L are subsets of a settheoretical language. The set of consequences of a theory T is called the set of theorems of T. It is assumed that the data DAT of a theory T are a subset of the axioms of T. A a theory has to be consistent.
Applying the concept of a Minimal Theoretical Framework to semiotic as it is e.g. introduced by Charles Morrris one could identify the formal structure of the theory as that part which Morris has called pure semiotic. A semiotic constructed along the lines of a Minimal Theoretical Framework would also be a deductive system. Pure semiotic would be transformed into descriptive semiotic if one combines the formal structure with methods of measurement and some data-protocols. Descriptive Semiotic is created by the application of pure semiotic to some supposed domain of investigation constituted by defined methods of measurement.
Comments are welcomed to doeb@inm.de
Daimlerstrasse 32, D-60314 Frankfurt am Main, Tel +49-69-941963-10, Fax +49-69-941963-22